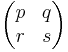Elliptic function
In complex analysis, an elliptic function is a function defined on the complex plane that is periodic in two directions (a doubly periodic function) and at the same time is meromorphic. Historically, elliptic functions were discovered as inverse functions of elliptic integrals; these in turn were studied in connection with the problem of the arc length of an ellipse, whence the name derives.
Contents |
Definition
Formally, an elliptic function is a meromorphic function f defined on C for which there exist two non-zero complex numbers a and b with a/b not real, such that
- f(z + a) = f(z + b) = f(z) for all z in C
wherever f(z) is defined. From this it follows that
- f(z + ma + nb) = f(z) for all z in C and all integers m and n.
There are two methods of constructing 'canonical' elliptic functions: those of Jacobi and Weierstrass. In the theory, modern authors mostly follow Karl Weierstrass: the notations of Weierstrass's elliptic functions based on his 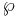 -function are convenient, and any elliptic function can be expressed in terms of these. However it is the functions of Jacobi that appear most commonly in practical problems, especially the need to avoid complex numbers, having a mapping from real to real, where the imaginary part is unnecessary or physically insignificant. Weierstrass became interested in these functions as a student of Christoph Gudermann, a student of Carl Friedrich Gauss.
-function are convenient, and any elliptic function can be expressed in terms of these. However it is the functions of Jacobi that appear most commonly in practical problems, especially the need to avoid complex numbers, having a mapping from real to real, where the imaginary part is unnecessary or physically insignificant. Weierstrass became interested in these functions as a student of Christoph Gudermann, a student of Carl Friedrich Gauss.
The elliptic functions introduced by Jacobi, and the auxiliary theta functions (not doubly periodic), are more complicated but important both for the history and for general theory. The primary difference between these two theories is that the Weierstrass functions have second-order and higher-order poles located at the corners of the periodic lattice, whereas the Jacobi functions have simple poles. The development of the Weierstrass theory is easier to present and understand, having fewer complications.
More generally, the study of elliptic functions is closely related to the study of modular functions and modular forms, a relationship proven by the modularity theorem. Examples of this relationship include the j-invariant, the Eisenstein series and the Dedekind eta function.
Properties
- Any complex number ω such that f(z + ω) = f(z) for all z in C is called a period of f. If the two periods a and b are such that any other period ω can be written as ω = ma + nb with integers m and n, then a and b are called fundamental periods. Every elliptic function has a fundamental pair of periods, but this pair is not unique, as described below.
- If a and b are fundamental periods describing a lattice, then exactly the same lattice can be obtained by the fundamental periods a' and b' where a' = p a + q b and b' = r a + s b where p, q, r and s being integers satisfying p s − q r = 1. That is, the matrix
-
- has determinant one, and thus belongs to the modular group. In other words, if a and b are fundamental periods of an elliptic function, then so are a' and b' .
- If a and b are fundamental periods, then any parallelogram with vertices z, z + a, z + b, z + a + b is called a fundamental parallelogram. Shifting such a parallelogram by integral multiples of a and b yields a copy of the parallelogram, and the function f behaves identically on all these copies, because of the periodicity.
- The number of poles in any fundamental parallelogram is finite (and the same for all fundamental parallelograms). Unless the elliptic function is constant, any fundamental parallelogram has at least one pole, a consequence of Liouville's theorem.
- The sum of the orders of the poles in any fundamental parallelogram is called the order of the elliptic function. The sum of the residues of the poles in any fundamental parallelogram is equal to zero, so in particular no elliptic function can have order one.
- The number of zeros (counted with multiplicity) in any fundamental parallelogram is equal to the order of the elliptic function.
- The set of all elliptic functions which share some two periods form a field.
- The derivative of an elliptic function is again an elliptic function, with the same periods.
- The Weierstrass elliptic function ℘ is the prototypical elliptic function, and in fact, the field of elliptic functions with respect to a given lattice is generated by ℘ and its derivative ℘′.
See also
References
- Abramowitz, Milton; Stegun, Irene A., eds. (1965), "Chapter 16", Handbook of Mathematical Functions with Formulas, Graphs, and Mathematical Tables, New York: Dover, pp. 567, ISBN 978-0486612720, MR0167642, http://www.math.sfu.ca/~cbm/aands/page_567.htm See also chapter 18. (only considers the case of real invariants).
- N. I. Akhiezer, Elements of the Theory of Elliptic Functions, (1970) Moscow, translated into English as AMS Translations of Mathematical Monographs Volume 79 (1990) AMS, Rhode Island ISBN 0-8218-4532-2
- Tom M. Apostol, Modular Functions and Dirichlet Series in Number Theory, Springer-Verlag, New York, 1976. ISBN 0-387-97127-0 (See Chapter 1.)
- E. T. Whittaker and G. N. Watson. A course of modern analysis, Cambridge University Press, 1952